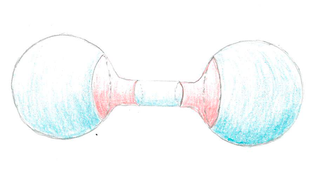
First introduced by Calabi, and also described by Cheeger in one of his famous papers, these dumbbell-shaped surfaces are a very well studied example in Spectral Geometry. The first eigenvalue of the Laplacian of these surfaces is proportional to the radius of the cylinder joining the two spheres. Thus, considering dumbbells with very thin cylinders, we obtain surfaces with arbitrarily small first eigenvalue.
In view of Lichnerowicz's theorem, this example shows that to find a positive lower bound for the first eigenvalue, one needs some kind of curvature assumption. Together with Kamryn Spinelli and Connor C. Anderson, two undergraduate students at Worcester Polytechnic Institute, we have been exploring this example in the context of integral curvature assumptions. Our exploration lead to a joint publication at PUMP Journal of Undergraduate Research, and the students presented our findings at the 2021 MAA MathFest and in the 2022 Joint Mathematics Meeting.
In view of Lichnerowicz's theorem, this example shows that to find a positive lower bound for the first eigenvalue, one needs some kind of curvature assumption. Together with Kamryn Spinelli and Connor C. Anderson, two undergraduate students at Worcester Polytechnic Institute, we have been exploring this example in the context of integral curvature assumptions. Our exploration lead to a joint publication at PUMP Journal of Undergraduate Research, and the students presented our findings at the 2021 MAA MathFest and in the 2022 Joint Mathematics Meeting.
 RSS Feed
RSS Feed
